تكامل مونت كارلو

An illustration of Monte Carlo integration. In this example, the domain D is the inner circle and the domain E is the square. Because the square's area (4) can be easily calculated, the area of the circle (π*1²) can be estimated by the ratio (0.8) of the points inside the circle (40) to the total number of points (50), yielding an approximation for the circle's area of 4*0.8 = 3.2 ≈ π*1².

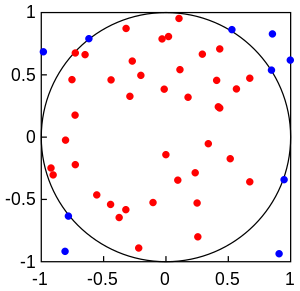
في الرياضيات، تكامل مونت كارلو (بالإنجليزية: Monte Carlo integration)‏ هي طريقة تكامل العددي تستخدم لإجراء التكامل العددي لدالة ما عن طريق أخذ قيم عشوائية من مجال الدالة.

انظر أيضا

• طريقة مونت كارلو