|
تضامنًا مع حق الشعب الفلسطيني |
اختبار كولموغوروف-سميرنوف
اختبار كولموغوروف-سميرنوف |
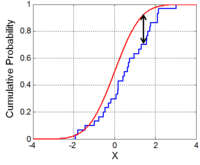
اختبار كولموجوروف-سميرنوف (بالإنجليزية: Kolmogorov-Smirnov test) اختبار إحصائي يُقارن توزيع المجتمع الإحصائي من خلال عينتين مستقلتين مأخوذتين من هذا المجتمع. ويمكن استخدامه لمقارنة أي توزيع نظري مع التوزيع المشاهد. يسمى في بعض الأحيان حسن المطابقة، وهو يحدث في بعض قوانين القياسات الببليومترية bibliometrics وفي الرياضيات والحقول الأخرى.
إحصائيات
يعطي المؤلفون الصيغة التالية:[1]
D = Maximum |F1(X) - F2(X)|
حيث:
F1(X) هي التوزيع التكراري المتجمع cummulative frequency distribution للعينة الأولى عند القيمة X
F2(X) هي التوزيع التكراري المتجمع cummulative frequency distribution للعينة الثانية عند القيمة X
|| هي عملية القيمة المطلقة
كما نشر Cook الدراسة التالية وتبعها بخطاب إلى المحرر:[2][3]
وفيها استخدم Cook اختبار Kolmogorov-Smirnov test لتحديد مدى توافق البيانات المشاهدة عن إنتاج أشهر القطع الموسيقية بواسطة الفنانين مع البيانات النظرية في قانون لوتكا Lotka's law وقانون برادفورد Bradford's law كما قام Budd and Seavey (1990) باستخدام Kolmogorov-Smirnov test لتقييم قانون لوتكا Lotka's law لدراسة التأليف في حقل المكتبات.[4]
روابط خارجية
- Hazewinkel، Michiel، المحرر (2001)، "Kolmogorov–Smirnov test"، Encyclopedia of Mathematics، سبرنجر، ISBN:978-1-55608-010-4
- Short introduction
- KS test explanation
- JavaScript implementation of one- and two-sided tests
- Online calculator with the KS test
- Open-source C++ code to compute the Kolmogorov distribution and perform the KS test
- Paper on Evaluating Kolmogorov’s Distribution; contains C implementation. This is the method used in ماتلاب.
- Paper powerlaw: A Python Package for Analysis of Heavy-Tailed Distributions; Jeff Alstott, Ed Bullmore, Dietmar Plenz. Among others, it also performs the Kolmogorov–Smirnov test. Source code and installers of powerlaw package are available at PyPi.
المراجع
- ^ Freed, M. N., Hess, R. K., and Ryan, J. M. (1989). The educator's desk reference. New York: Macmillan.
- ^ Cook, K. L. (1989). Laws of scattering applied to popular music. Journal of the American Society for Info rmation Science, 40, 277-283.
- ^ Cook, K. L. (1991). [Letter to the editor]. Journal of the American Society for Information Science, 42, 113-114.
- ^ Budd, J. M. and Seavey, C. A. (1990). Characteristics of journal authorship by academic librarians. College & Research Libraries, 51, 463-470.